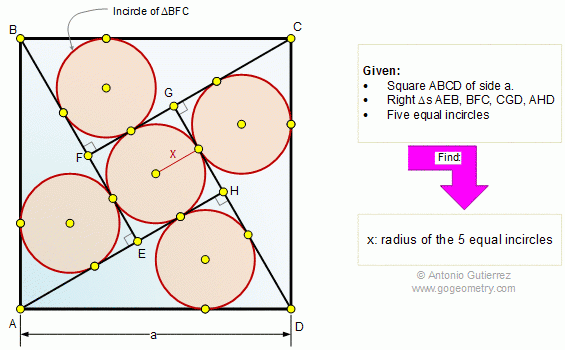
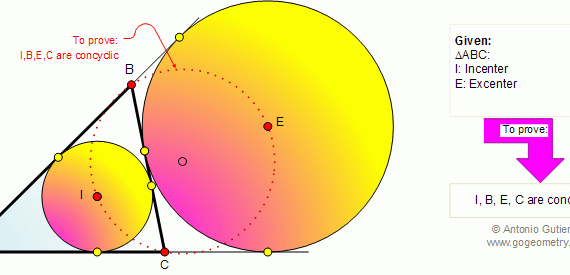
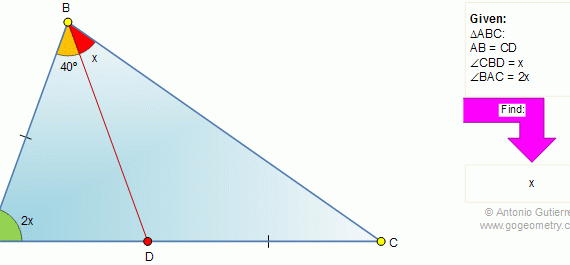
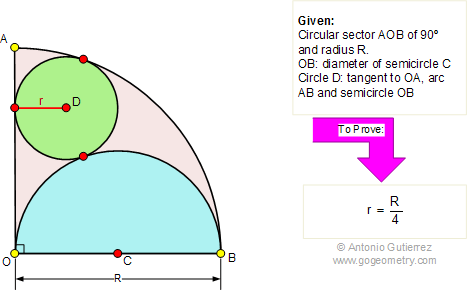
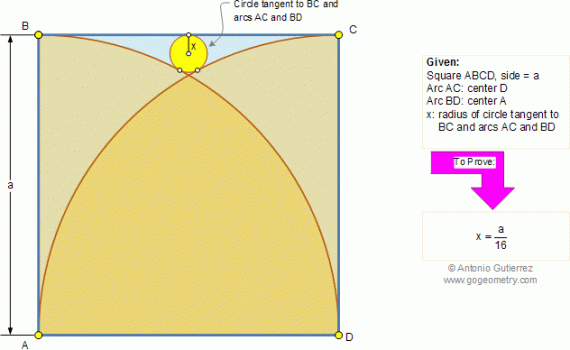
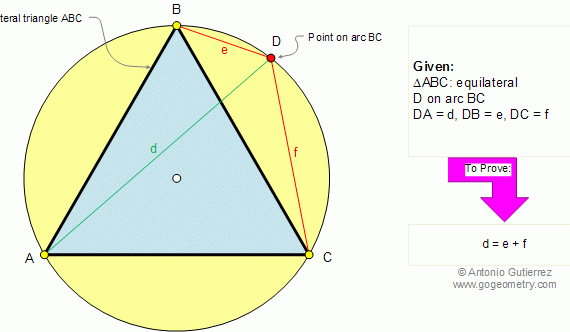
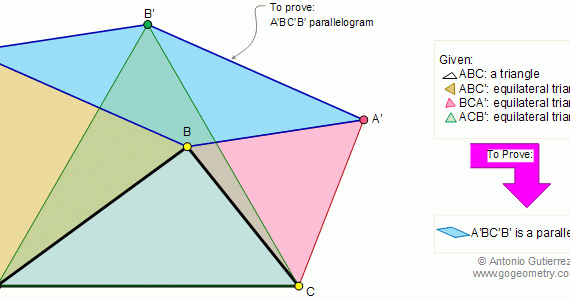
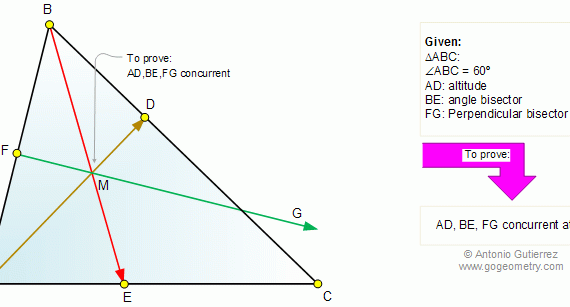
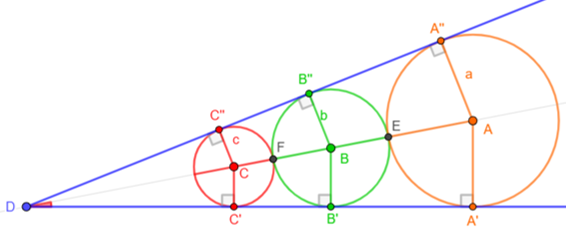
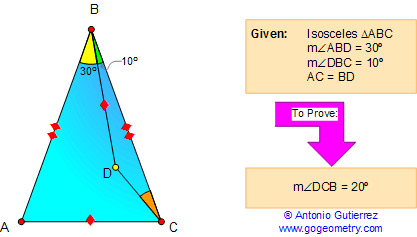
From Gogeometry Using Additional points congruent triangles Equilateral triangle Area of a triangle •ABCDEF is a regular hexagon with center O and side c => AB//DE, BC//EF et CD//FA •Let R=OA=OB=OC=OD=OE=OF •Define G such as PG⊥ AB. The same for H,I,J,K,L •AOF is an equilateral triangle with height h, such […]

Gogeometry Problem 983

From Gogeometry Using Pythagoras congruent triangles Similar triangles Equilateral triangle Area of a triangle Tangents to a circle Inradius in right triangle •Pythagoras on triangle ΔBFC => (1) BF^2+CF^2=BC^2=a^2 •Incircle in a right triangle => (2) 2x=BF+CF-BC =BF+CF-a •[ABCD]= 4 triangles areas + square with side 2x => (3) S=a^2=2BF.CF+4x^2 […]

Gogeometry Problem 491

From Gogeometry Using central and inscribed angle congruent triangles Concyclic points Tangents to a circle Inscribed angle and tangent to a circle Inradius in right triangle •Point I is on the intersection of the internal angle bisector•=> AI and IB are internal angle bisector •Point O is on the intersection […]

Gogeometry Problem 623

From Gogeometry Using Additional points Inscribed angles in a circle congruent triangles Concyclic points

Gogeometry Problem 412

From Gogeometry Using Area of a triangle Tangents to a circle Inscribed circle of a triangle b=ACAN=AM, AF=AE, MF=NE,CP=CO, CF=CG, PF=OG,BE=BGr=DF=DE=DGx=JM=JN=LO=LPMP=2x S=[ABC]=r(AB+BC+AC)/2 => S=r(AB+CB+b)/2 AB=AE+EB=AF+EBCB=CG+GB=CF+GBAB+CB=AF+CF+EB+GB=b+2BE=> (1) S=r(b+BE) But S=[AMJN]+[MFDENJ]+[PFDGOLP]+[EBGD]S=xAM+(r+x)MF+xCP+(r+x)PF + rEB, knowing that [EBGD]=(rEB+rBG)/2=rEBS=x(AM+MF+CP+PF)+r(MF+PF) + rEB(2) S=xb+2xr + rEB (1) and (2) => S=r(b+BE)=xb+2xr+ rEBbr=xb+ 2xr Therefore x=br/(b+2r)

Gogeometry Problem 418

Using Additional points congruent triangles Area of a triangle [OQL]=OL . KL/2=bc/2[OPQ]+[LKQ]=PQ . KL/2+QK.KL/2=(PQ+QK).KL/2=PK.KL=bc/2=>[OQL]=[OPQ]+[LKQ]=bc/2[OPKL]= [OQL]+[OPQ]+[LKQ]=2bc/2=bc In the same way [LMHI]= [IJEF]= [FGNO]= [OPKL]= bc[QRST]= [OLIF] + [OQL] + [LRI] + [ISF] + [FTO][QRST]= c^2+2bc=c(c+2b) Therefore [QRST]= ac (Situation A) But, this is not true if b is too big, then […]

HP008

From Gogeometry Define a=AB=BC=AC DC and DB tangents to Circle O1 => DG=DE=bAD and AB tangents to Circle O1 => AG=AJ=dDB and BA tangents to Circle O1 => BE=BJ=c DB and BC tangents to Circle O2 => BF=BK=eDC and BC tangents to Circle O2 => CI=CK=f a=d+c=e+fDF=b+c+e, DI=b+d+a+fDC and DB […]

Gogeometry Problem 1372

From Gogeometry Using : Additional points Inscribed angles in a circle central and inscribed angle Tangents to a circle Inscribed angle and tangent to a circle Without using : Law of cosines Define : F the tangent intersection of CirleD and CircleO E intersection of CircleD and OA G intersection […]

Gogeometry Problem 283

From Gogeometry Using : Inscribed angles in a circle central and inscribed angle congruent triangles Similar triangles Tangents to a circle Inscribed angle and tangent to a circle   Without using : Pythagoras Define G the center of CircleG with radius x Define the point E as the only point […]

Gogeometry Problem 276

From Gogeometry Using : Isometric transformation Additional points congruent triangles Define α = ∠BAF and β = ∠DAE α+β+45°=90° => α+β = 45° Define E’ rotation of E anticlockwise with an angle of 90° =>AE=AE’, β = ∠DAE = ∠BAE’ ∠FAE’= α+β = 45° ΔFAE’ is congruent to ΔFAE (SAS) […]

Gogeometry Problem 1076

From Gogeometry   Using : Additional points Inscribed angles in a circle congruent triangles Equilateral triangle CAD intercepts arc CD such as CBD => ∠CAD= ∠CBD Define D’ n AD such as AD’C is congruent to BDC => AD’=f and ∠ACD’= ∠BCD ∠ACD= ∠ACB+ ∠BCD=Π/3+ ∠BCD And ∠ACD= ∠ACD’+ ∠D’CD=∠BCD+ […]

Gogeometry Problem 256

From Gogeomety     Using : Equilateral triangle Congruent triangles Parallelogram ΔACB’ and ΔBCA’ are equilateral => ∠ACB’ =∠BCA’ = Π/3 ∠ACB = ∠ACB’ – ∠B’CB = Π/3 – ∠B’CB ∠B’CA’ = ∠BCA’ – ∠B’CB = Π/3 – ∠B’CB =>∠ACB =∠B’CA’ ΔACB is congruent to ΔB’CA’ (SAS) => BA=B’A’ In […]

Gogeometry Problem 242

From Gogeometry Using congruent triangles Similar triangles •AD altitude => ∠BDA = π/2 •BE angle bisector =>∠ABE=∠CBE= π/6 •Define M as the intersection of BE and AD •Define F on AB such as BF ⊥ FM •Then we have to demonstrate that FB=FA to prove that FG is perpendicular bisector […]

Gogeometry Problem 663

Gogeometry Problem 315 From Gogeometry Using Similar triangles Define n=CDΔBDB’ is similar to ΔCDC’ => BD/BB’=CD/CC’=>(b+c+n)/b=n/c => b+c+n=bn/c => n(b/c-1)=b+c=> (1)  n=c(b+c)/(b-c)ΔADA’ is similar to ΔCDC’ => AD/AA’=CD/CC’=>(a+2b+c+n)/a=n/c => ac+2bc+c^2+nc=an => n(a-c)= ac+2bc+c^2=> n= (ac+2bc+c2)/(a-c) => n= c(a+2b+c)/(a-c) (2)(1)&(2) : (b+c)/(b-c)= (a+2b+c)/(a-c)=>(b+c)(a-c)=(a+2b+c)(b-c)=>ab-bc+ac-c^2=ab-ac+2b^2-2bc+bc-c^2=> 2ac=-2c^2=>ac=c^2

Gogeometry Problem 315

From Gogeometry Using Inscribed angles in a circle Equilateral triangle Concyclic points ∠BAD= π/2 => B, A and D are concyclic, circle with center F middle of BD=> FB=FA=FD and ∠BAF=15°In the same way, GC=GA=GE and ∠CAG=15°=>∠FAG=90-15-15=60°ΔFAG is isosceles in A (AF=AG) and ∠FAG=60° => ΔFAG is equilateral Therefore x=FG=BD/2=CE/2=1

Gogeometry Problem 406

From Gogeometry Using Pythagoras central and inscribed angle Equilateral triangle DC=DA and ∠ADC= π/3 => ΔADC is equilateral∠CAD= π/3, ∠BAC+∠CAD=∠BAD=75° => ∠BAC=15°∠ACD= π/3, ∠BCA+∠ACD=∠BCD=155° => ∠BCA=75°∠BAC=15° et ∠BCA=75° => ∠ABC= π/2 ΔADC is equilateral and EC=ED => ∠AEC= π/2∠ABC= π/2 et ∠AEC= π/2 => A, B, C and E are […]

Gogeometry Problem 405

From Gogeometry Using Additional points congruent triangles Equilateral triangle Thales theorem ∠ABC = 30°+10°=40°Define F on AC and on the angle bisector of <ABCΔABC isosceles in B => ∠ABF=∠FBC = 40°/2=20°Define I such as ΔAIC is equilateral => ∠IAC=60°ΔABC isosceles in B => ∠ABI=∠CBI = 20°∠ABC = 40° and ΔABC […]

Gogeometry Problem 60

Definition : In euclidean geometry, a kite is a quadrilateral whose four sides can be grouped into two pairs of equal-length sides that are adjacent to each other. Property : Every kite is orthodiagonal, meaning that its two diagonals are at right angles to each other

Kite